
As usual on Options education, we will use information from the Investopedia Staff.
As more and more traders have learned of the multitude of potential benefits available to them via the use of options, the trading volume in options has proliferated over the years. This trend has also been driven by the advent of electronic trading and data dissemination. Some traders use options to speculate on price direction, others tohedge existing or anticipated positions and others still to craft unique positions that offer benefits not routinely available to the trader of just the underlying stock, index or futures contract (for example, the ability to make money if the underlying security remains relatively unchanged). Regardless of their objective, one of the keys to success is to pick the right option, or combination of options, needed to create a position with the desired risk-to-reward tradeoff(s). As such, today's savvy option trader is typically looking at a more sophisticated set of data when it comes to options than the traders of decades past.
The Old Days of Option Price Reporting
In "the old days" some newspapers used to list rows and rows of nearly indecipherable option price data deep within its financial section such as that displayed in Figure 1.
 |
| Figure 1: Option data from a newspaper |
Investor's Business Daily and the Wall Street Journal still include a partial listing of option data for many of the more active optionable stocks. The old newspaper listings included mostly just the basics – a "P" or a "C" to indicate if the option a call or a put, the strike price, the last trade price for the option, and in some cases, volume and open interest figures. And while this was all well and good, many of today's option traders have a greater understanding of the variables that drive option trades. Among these variables are a number of "Greek" values derived from an option pricing model, implied option volatility and the all important bid/ask spread. (Learn more in Using the Greeks to Understand Options.)


As a result, more and more traders are finding option data via on-line sources. While each source has its own format for presenting the data, the key variables generally include those listed in Figure 2. The option listing shown in Figure 2 is from Optionetics Platinum software. The variables listed are the ones most looked at by today's better educated option trader.
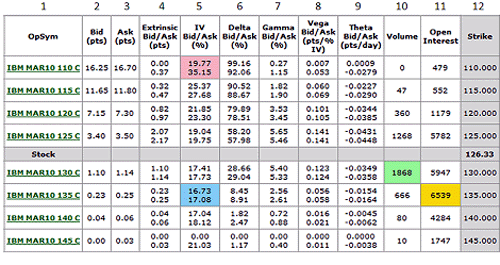 |
| Figure 2: March call options for IBM |
The data provided in Figure 2 provides the following information:
Column 1 – OpSym: this field designates the underlying stock symbol (IBM), the contract month and year (MAR10 means March of 2010), the strike price (110, 115, 120, etc.) and whether it is a call or a put option (a C or a P).
Column 2 – Bid (pts): The "bid" price is the latest price offered by a market maker to buy a particular option. What this means is that if you enter a "market order" to sell the March 2010, 125 call, you would sell it at the bid price of $3.40.
Column 3 – Ask (pts): The "ask" price is the latest price offered by a market maker to sell a particular option. What this means is that if you enter a "market order" to buy the March 2010, 125 call, you would buy it at the ask price of $3.50.
NOTE: Buying at the bid and selling at the ask is how market makers make their living. It is imperative for an option trader to consider the difference between the bid and ask price when considering any option trade. The more active the option, typically the tighter the bid/ask spread. A wide spread can be problematic for any trader, especially a short-term trader. If the bid is $3.40 and the ask is $3.50, the implication is that if you bought the option one moment (at $3.50 ask) and turned around and sold it an instant later (at $3.40 bid), even though the price of the option did not change, you would lose -2.85% on the trade ((3.40-3.50)/3.50).
Column 1 – OpSym: this field designates the underlying stock symbol (IBM), the contract month and year (MAR10 means March of 2010), the strike price (110, 115, 120, etc.) and whether it is a call or a put option (a C or a P).
Column 2 – Bid (pts): The "bid" price is the latest price offered by a market maker to buy a particular option. What this means is that if you enter a "market order" to sell the March 2010, 125 call, you would sell it at the bid price of $3.40.
Column 3 – Ask (pts): The "ask" price is the latest price offered by a market maker to sell a particular option. What this means is that if you enter a "market order" to buy the March 2010, 125 call, you would buy it at the ask price of $3.50.
NOTE: Buying at the bid and selling at the ask is how market makers make their living. It is imperative for an option trader to consider the difference between the bid and ask price when considering any option trade. The more active the option, typically the tighter the bid/ask spread. A wide spread can be problematic for any trader, especially a short-term trader. If the bid is $3.40 and the ask is $3.50, the implication is that if you bought the option one moment (at $3.50 ask) and turned around and sold it an instant later (at $3.40 bid), even though the price of the option did not change, you would lose -2.85% on the trade ((3.40-3.50)/3.50).
Column 4 – Extrinsic Bid/Ask (pts): This column displays the amount of time premium built into the price of each option (in this example there are two prices, one based on the bid price and the other on the ask price). This is important to note because all options lose all of their time premium by the time of option expiration. So this value reflects the entire amount of time premium presently built into the price of the option.
Column 5 – Implied Volatility (IV) Bid/Ask (%): This value is calculated by an option pricing model such as the Black-Scholes model, and represents the level of expected future volatility based on the current price of the option and other known option pricing variables (including the amount of time until expiration, the difference between the strike price and the actual stock price and a risk-free interest rate). The higher the IV Bid/Ask (%)the more time premium is built into the price of the option and vice versa. If you have access to the historical range of IV values for the security in question you can determine if the current level of extrinsic value is presently on the high end (good for writing options) or low end (good for buying options).
Column 6 – Delta Bid/Ask (%): Delta is a Greek value derived from an option pricing model and which represents the "stock equivalent position" for an option. The delta for a call option can range from 0 to 100 (and for a put option from 0 to -100). The present reward/risk characteristics associated with holding a call option with a delta of 50 is essentially the same as holding 50 shares of stock. If the stock goes up one full point, the option will gain roughly one half a point. The further an option is in-the-money, the more the position acts like a stock position. In other words, as delta approaches 100 the option trades more and more like the underlying stock i.e., an option with a delta of 100 would gain or lose one full point for each one dollar gain or loss in the underlying stock price. (For more check out Using the Greeks to Understand Options.)
Column 7 – Gamma Bid/Ask (%): Gamma is another Greek value derived from an option pricing model. Gamma tells you how many deltas the option will gain or lose if the underlying stock rises by one full point. So for example, if we bought the March 2010 125 call at $3.50, we would have a delta of 58.20. In other words, if IBM stock rises by a dollar this option should gain roughly $0.5820 in value. In addition, if the stock rises in price today by one full point this option will gain 5.65 deltas (the current gamma value) and would then have a delta of 63.85. From there another one point gain in the price of the stock would result in a price gain for the option of roughly $0.6385.
Column 8 – Vega Bid/Ask (pts/% IV): Vega is a Greek value that indicates the amount by which the price of the option would be expected to rise or fall based solely on a one point increase in implied volatility. So looking once again at the March 2010 125 call, if implied volatility rose one point – from 19.04% to 20.04%, the price of this option would gain $0.141. This indicates why it is preferable to buy options when implied volatility is low (you pay relatively less time premium and a subsequent rise in IV will inflate the price of the option) and to write options when implied volatility is high (as more premium is available and a subsequent decline in IV will deflate the price of the option).
Column 9 – Theta Bid/Ask (pts/day): As was noted in the extrinsic value column, all options lose all time premium by expiration. In addition, "time decay" as it is known, accelerates as expiration draws closer. Theta is the Greek value that indicates how much value an option will lose with the passage of one day's time. At present, the March 2010 125 Call will lose $0.0431 of value due solely to the passage of one day's time, even if the option and all other Greek values are otherwise unchanged.
Column 10 – Volume: This simply tells you how many contracts of a particular option were traded during the latest session. Typically – though not always - options with large volume will have relatively tighter bid/ask spreads as the competition to buy and sell these options is great.
Column 11 – Open Interest: This value indicates the total number of contracts of a particular option that have been opened but have not yet been offset.
Column 12 – Strike: The "strike price" for the option in question. This is the price that the buyer of that option can purchase the underlying security at if he chooses to exercise his option. It is also the price at which the writer of the option must sell the underlying security if the option is exercised against him.
A table for the respective put options would similar, with two primary differences:
- Call options are more expensive the lower the strike price, put options are more expensive the higher the strike price. With calls, the lower strike prices have the highest option prices, with option prices declining at each higher strike level. This is because each successive strike price is either less in-the-money or more out-of-the-money, thus each contains less "intrinsic value" than the option at the next lower strike price. With puts, it is just the opposite.
- As the strike prices go higher, put options become either less-out-of-the-money or more in-the-money and thus accrete more intrinsic value. Thus with puts the option prices are greater as the strike prices rise. For call options, the delta values are positive and are higher at lower strike price. For put options, the delta values are negative and are higher at higher strike price. The negative values for put options derive from the fact that they represent a stock equivalent position. Buying a put option is similar to entering a short position in a stock, hence the negative delta value.
Option trading and the sophistication level of the average option trader have come a long way since option trading began decades ago. Today's option quote screen reflects these advances.
Click on the link below.
Click on the link below.
Get a free book on Options Trading click the link above.
No comments:
Post a Comment